Golden Ratio¶
687 words
Last October I already went on about the golden ratio and how people brabble about it all along – particularly if they don’t even have the slightest idea what they are talking about. I was particularly astonished by the tendency of people to equate the ‘rule of thirds’ with the golden ratio. Which I think is wrong for (at least) two reasons.
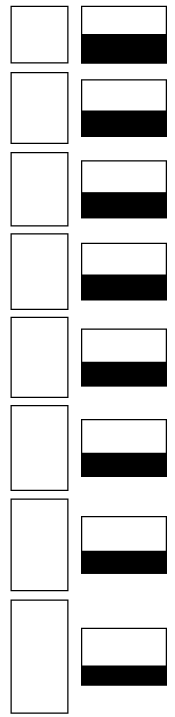 The first – and most trivial – reason is the maths: Those numbers obviously aren’t the same. When using the ‘rule of thirds’ you get one piece which is one third of the total length and another which covers the remaining two thirds. That is, the longer one is twice the length of the shorter one. Whereas when using the golden ratio instead the factor would be the ‘golden ratio’ ϕ = ½(1+√5) ≈ 1,618. These are quite different numbers.
The first – and most trivial – reason is the maths: Those numbers obviously aren’t the same. When using the ‘rule of thirds’ you get one piece which is one third of the total length and another which covers the remaining two thirds. That is, the longer one is twice the length of the shorter one. Whereas when using the golden ratio instead the factor would be the ‘golden ratio’ ϕ = ½(1+√5) ≈ 1,618. These are quite different numbers.
It’s probably best to look at their ratio to get an idea about how different they are: ϕ/2 ≈ 0,81, which is quite a bit away from being the same (i.e. 1). It may be instructive to think about other ratios to drive this point home. A 35mm photo has a 3:2 aspect ratio, while most consumer digital cameras have a 4:3 aspect ratio. Most people will agree that those ratios look very different. Yet, comparing these – (4/3)/(3/2) = 8/9 ≈ 0,89 – reveals that their aspect ratios are actually much more similar than the ones compared above. Another interesting comparison might be that between A-paper sizes which tend to look very tall with their √2 ≈ 1,41 aspect ratio and US-letter paper which looks much more friendly with its aspect ratio of about 1,29 (27,94cm by 21,59cm). Compare these once more and you’ll get 1,29/√2 ≈ 0,91 – another number which suggests that A4 and US letter paper are much more similar in their aspect ratios than ‘rule of thirds’ and the golden ratio are.
I hope that these examples help to illustrate the dry numbers a bit. Most people will agree that digital and analogue photos have very different aspect ratios. And most people will agree that US letter and A4 paper have a very different feel to them. Yet, people find it hard to acknowledge that the golden ratio is very different from the ‘rule of thirds’ while – just going by the numbers – their difference is much more than in the other examples.
The second reason I see is a more conceptual one. Applying the ‘rule of thirds’ is very simple. All it requires you to do when splitting up a length is to divide it a third into its length. Thinking about the golden ratio is much more involved and subtle: You either want to make sure that the ratio between the shorter and the longer part equals the ratio between the longer part and the whole length – a/b = b/(a+b) for the maths buffs. Alternatively, it can be helpful to think about pentagrams which have golden ratios built into them pretty much everywhere. I’d say that doing this requires a completely different mindset and level of attention than applying the ‘rule of thirds’ does.
People like to shrug when I get worked up on this issue. Maths-inclined people of course get the point with the numbers but could hardly care less about the actual use of this. While arty (and stereotypically maths hating) people can’t be asked to even look at the numbers. Hence the more concrete examples above. Perhaps they help.
In fact, I even found a book at the library on the topic. It’s an old book called Der Goldene Schnitt which isn’t too mathematical and comes with both constructions plus plenty of historical examples from architecture or art and even with book layout examples.
[The image accompanying the beginning of the text shows numbers as aspect ratios on the left and as ratios of the whole at the right. The numbers used are, from top to bottom: 1, 1,25 (5/4), 1,29 (US Letter), 1,33 (4/3), 1,41 (A-paper), 1,5 (3/2), 1,62 (golden ratio), 2 (rule of thirds).]

